Today, we practiced more antiderivative questions using "Integration by Parts".
Here we go...
1. Find the antiderivatives of the following:
 Q: How can we decide which function should be "u" (aka "f") and which one should be "dv" (aka "g' ")??
Q: How can we decide which function should be "u" (aka "f") and which one should be "dv" (aka "g' ")??A: Good question! Today, we learned a new "rule". It's the...

This rule is used to decide which of the two functions will be "f" and "g' ". This rule is followed from top to bottom (or left to right if you wrote it in that type of manner). For example, if an "algebraic" and a "trigonometric" is present in the problem, then "f"= "algebraic" and "g' "= "trigonometric". According to the rule, "A" comes before "T".
Moving on...

 Q: How come we did the "Integration by Parts" twice?
Q: How come we did the "Integration by Parts" twice?A: We did the technique twice because we dont know the antiderivative of "xcosx". We have to use the technique again to find the antiderivative of this expression.
Then, we answered some trig problems...
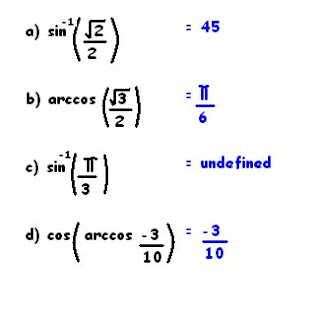 Note: "Arc(cos or sin)" is the same as "sin or cos"^-1.
Note: "Arc(cos or sin)" is the same as "sin or cos"^-1.The answer to letter "c" is undefined because the value is greater than 1. The answer to letter "d" is -3/10 because cos and arccos cancel each other out.
Finally we looked at this graph...
 This is the graph of y= Sine. It is fixed in the domain, (-pi/2, pi/2). By doing this, the graph of sine is now a "One-to-one" function. It means that each input has 1 and only 1 output.
This is the graph of y= Sine. It is fixed in the domain, (-pi/2, pi/2). By doing this, the graph of sine is now a "One-to-one" function. It means that each input has 1 and only 1 output.Refresher:
1. Vertical Line Test- this test is used to determine if the graph is a function or a relation. If the line test touches the graph more than twice (1 input has 2 or more outputs), then it is a relation.
2. Horizontal Line Test- this test is used to determine if the inverse of a function is a function or a relation. If the line touches the graph of the parent function more than twice, then the inverse of the graph is a relation.
I hope I covered most of the things we did for this class. XD
Next scribe will be Danny.









 .
. 

















 This graph is the same as the one up there, except that the axes are now labeled.
This graph is the same as the one up there, except that the axes are now labeled. 
