Ben Saunders

Arctic explorer Ben Saunders recounts his harrowing solo journey to the North Pole, complete with gorgeous images, amusing anecdotes and previously unseen video footage from the Pole. At 26, Saunders became the youngest person ever to ski solo to the North Pole, updating his blog daily during the trek. He's now planning the next journey, SOUTH, an unprecedented, roundtrip expedition across Antarctica and back.

His story is spellbinding. There's a really important message for you in the last two minutes ... but you won't really understand it unless you watch the whole thing.

Click on the picture. (18 min. 48 sec.)

Bono - TED Prize Winner

For the past 20 years, members of the TED community have gathered together to share ideas and passions that are big enough to change the world. Each year they will honor a maximum of three individuals who have shown that they can, in some way, positively impact life on this planet.

Rather than simply receiving financial support, winners of the TED Prize are granted something extraordinary: something which children dream about, but which adults assume is merely the stuff of fairy-tales.

They are granted three WISHES to change the world.

They may wish for anything. And TED will seek to make their wishes come true.

Bono won the TED prize in 2005.

Rock star and activist Bono accepts the 2005 TEDPrize with a riveting talk about our moral obligation (and economic incentive) to help lift Africa out of poverty. He unveils his TEDPrize wishes by challenging the TED community to help build a social movement of more than one million American activists for Africa; to tell people one billion times about the ONE campaign; and to connect every hospital, health clinic, and school in one African country, Ethiopia, to the Internet.

Click on the picture. (28 min. 37 sec.)



For more: Read the update on Bono's wishes.

Richard St. John

Why do people succeed? Richard St. John compacts seven years of research into an unmissable 3-minute slideshow on the real secrets of success (Hint: Passion, persistence, and pushy mothers help).

Click on the picture. (3 min. 40 sec.)

Gregory Colbert

Photographer Gregory Colbert shares the remarkable images and film footage from his exhibit, "Ashes and Snow," and announces his founding of the Animal Copyright Foundation, which will require that royalties be paid when images of nature are used for commercial purposes.

The pictures are just stunning.

Click on the picture. (18 min. 42 sec.)

Hans Rowling

Hans Rowling is a professor of of international health at Sweden's world-renowned Karolinska Institute.

Watch how he displays and talks about statistics. He'll make you laugh and he'll make you think.

Click on the picture. (20 min. 35 sec.)

Pi and e ... their first date

You know enough math to get all the jokes in this now ... ;-)



Have an excellent holiday!

Cheers,
Mr. K.

Flickr Assignment Rubric v1.0 - We're Out of Beta!

Here is the rubric we've settled on together.

Thanks to everyone who helped put this together. I found this to be a great experience for me as a teacher I hope it was also valuable to you as a student.

Anyway, here it is, version 1.0 fresh out of beta. ;-)

---

Flickr Assignment Rubric
It is paramount that the picture be in tune with the purpose of the assignment. It should show, first of all, the student's understanding of how the photo is related to mathematics. The hot spots are important too, because that's essentially your way of teaching other people. Creativity is a factor, because keeping one's interest in the photo contributes to the learning process. Finally, the picture quality should be kept in mind too. If we can't see the picture, it's going to be hard achieving all the other requirements.

Tags

The picture must be tagged properly with the course tag and assignment tag. If tags are misspelled or no tags are present the photo cannot be graded and will receive a grade of ZERO. Not tagging your photo properly and accurately is analogous to not handing in your work or not putting your name on it.

Classification	Mathematical Content (50%)	Hot Spots (35%)	Photograph (15%)
Level 4	Packed with mathematical concepts/facts. (Minimum 7 concepts/facts.)	All hot spots accessible; i.e. "smaller" hot spots are "on top" of larger ones, they do not obscure each other. All hot spots are actually labels and relate to parts of the photo (not on blank space with filled in notes). One or more hot spots include a link to a relevant supporting resource on the internet. Minimum 7 hot spots.	In focus or appropriately focused for effect. The subject of the picture occurs "naturally," it is not a contrived shot. Really makes the viewer "see" math in a place they hadn't realized it existed. (Example: trigonometry)
Level 3	Significant number of concepts/facts included. (Minimum 5 concepts/facts.)	All hot spots accessible. Most hot spots are actually labels and relate to parts of the photo. Not more than one hot spot on blank space. One or more hot spots may include a link to a relevant supporting resource on the internet. Minimum 5 hot spots.	In focus or appropriately focused for effect. The subject of the photo has been "set up" or contrived yet still illustrates math found in "the real world." (Example: derivative)
Level 2	Some effort to include content evident. (Minimum 3 concepts/facts.)	Most hot spots accessible. Most hot spots are actually labels and relate to parts of the photo. More than one hot spot is on "blank" space. May or may not include links to relevant supporting resource on the internet. Minimum 3 hot spots.	In focus or appropriately focused for effect. Although it is a "real world" picture, objects have been used to "draw" the math. An obviously contrived shot. (Example: trigonometry)
Level 1	Very scarce content related to assignment.	Less than three hot spots are visible or have information related to the theme of the assignment.	It is evident that little effort went into finding and shooting a picture that reflects the theme of the assignment.
Level 0	Content unrelated to theme of assignment.	No hot spots or mostly unrelated to the theme of the assignment.	Out of focus and/or otherwise difficult to look at.

Creativity (up to 5% bonus)

The maximum possible mark for this assignment is 105%. You can earn up to 5% bonus marks for being creative in the way you approach this assignment. This is not a rigidly defined category and is open to interpretation. You can earn this bonus if your work can be described in one or more of these ways:

• unique and creative way of looking at the world, not something you'd usually think of;
• original and expressive;
  
• imaginative;
• fresh and unusual;
• a truly original approach.

First things first? One day left to go--

Wondering if you're having trouble putting first things first in these last days before winter break?

And

Wanting to wish each of you happiness, peace, and joy during this season! Be safe and enjoy!

Best,
Lani

bob

well this unit went by pretty fast. hmm i think that this unit was somewhat confussing. i got really confussed with the antiderivatives and especially the optimizaton problems. well i guess everythign else is okay. well good luck to me, i know i will need it

BOB

This unit went by extremely fast was alright in a way. We managed to get it done before the break which was the goal. Antiderivatives are a little tough but i found the optimization problems a little harder to understand. The easiest part of this unit would have to be the Mean Value Theorem, but even that provided a little bit of difficulty. The tough thing for me for optimization problems is finding the whole equations and everything, that's where I have the most trouble on. Well, anyways good luck too all on the test! I know we all basically expressed confusion and it was hectic on the pre-test but it's probably best to get this done now then to carry it on any longer.

BOB

This unit just flew by. I liked this unit, but when I did the pre-test I was like ... NOO! I completely blanked out and spent most of my time worrying about it.
I think the problem for me was the arithmetic part. I would get the right idea, but I would write the equation or something like that wrong. The optimization problems were alright i guess. I liked the mean value theorem. It was the easiest to understand. Antiderivatives were tricky. I found it alright. Mr. K explained it a WHOLE lot better than in the textbook. I'm not a textbook kinda person. I either get confused or can't get interested in it. The holidays played a part in my laziness this past unit. School just sort of drags on because you can't wait and thats BAD because thats when things get crazy! I had like three other tests to study for. It's like they all plan to make it like that- to get you all busy, worried, and crazy. And it doesn't help when people, like my bonkers family, getting even more crazy over stuff they should have did a long time ago. Kind of sounds familiar, doesn't it... XD. Good luck to you all and don't forget C.

BOB

Again, because the concept of antiderivatives is new to me, I'm still feeling my way through the problems. I'm not confident with my answers at all =(. I know that we have to apply the derivative rules and the relationships between f, f' and f", but sometimes I just don't know if I'm even on the right track. Maybe it'd help if we do the following:

(1) Review what we've done so far.
(2) Take notes
(3) Do the assigned problems
(4) Don't stress out =)

That's all for my bob!

BOB - PLUS C

Today was like walking in front of a wall... the pre-test was harder than I thought it would be. I was expecting optimization problems (which I kind of like) but there weren't any. My group took more time trying to calm down than actually solving the problems, but I did get number four on my own.. woohoo!!! However, I blanked out on the long answer. To be honest, I didn't do all of my homework for this unit. I mean come on, winter break is in three days... there's too many things to do on the list, who isn't excited? I thought this unit was going to be my favourite, but it got harder towards the end... those anti-derivatives... plus C!!! Hopefully, reading my notes and doing the questions I have is enough. Anyway, I should get a good night's sleep. Good luck everyone and HAPPY HOLIDAYS =)

bob

ahh it's that time again..I don't feel too good =/. Let's just get this over with. Well this test came really quick and I honestly think I'm not ready but hey I don't really have a choice. The pre-test went not so good because we had no idea how to do the long answer and we didn't get to discuss it which worries me for the test tomorrow. Also the multiple choice questions were quite difficult because my group pretty much guessed because even though we had done some work for the questions it did not match up with one of the possible answers which probably means we did it wrong. I think we should postpone the test till well never...haha I wish. Well I'm sure it'll be okay if I go study otherwise I have a feeling I won't do too good. At least I remembered to post this bob last minute, it almost slipped my mind but not I think i have guaranteed mark. woohoo...well good luck everyone cause I know I'll need it.

BOB

Well it's our last test before the winter break. This unit seems to be the easiest for me. Maybe because I'm doing more of my homework. It's great when I don't have to work! Well this chapter was about more applications on derivitives. Since we know a lot about derivitives already I guess that's why everbody finds this unit a bit easier. What I really liked was how we studied on word problems. I know I'm not particularly great in word problems, but it's like a break from all the numbers and crazy variables. They are included in the questions, however I like figuring out the picture. It's challenging, even though I usually get them kind of wrong :) But we did a lot of group problems that involved this kind of work. So I hope there's some on the test tomorrow. I reviewed some of the review questions and I only got half way. Hopefully I'll get to class really early to look at that answer book. I didn't really understand some of them, or parts of them. Well I guess it's time for some major studying again. My new goal is to review all my notes I did in that day so I won't forget it. That way it'll be new in my brain. It's difficult though, because it takes a lot of time. But I will try my very hardest!! GOOD LUCK EVERYONE, WE CAN DO IT!!! DONT FORGET TO ADD C. :D

BOB 5

Well, it's test time once again. I'm edging slightly towards holiday meltdown, so I don't think I'm in quite the right frame of mind to write this test, but that can't be helped. This is just a hard time of year for everyone, I think. I do feel that I understood most of the unit, though I find the optimization problems difficult, and I seem to need to see how to solve each specific type of problem, before I can solve it myself. It's generally the same sort of difficulty that I ran into with the word problems from last unit. Though I spent last unit focusing on those problems as they were my weakness, and there wasn't even one on the test! So I don't know what to do, there weren't any optimization problems or antiderivatives on the pretest. I don't know what I should focus on... And speaking of antiderivatives. I believe I have a good grasp of the idea behind them, but it is true that they are quite a lot harder than differentiating. I think they are the kind of thing that you just need to practice until the patterns become more familiar. And I hate it when stuff just doesn't exist.. imagine getting stuck on that for half an hour if your answer is impossible to find! And THANK YOU to Katrin for mentioning plus C in her bob, I was starting to forget about it. Well, I wish everyone luck and a nice relaxing vacation.

BOB #5

This chapter 5 unit on More Applications on the Derivative helped me understand the concept with derivatives a lot more. At first I never knew that you can use a number line to figure where there are max and mins and global mins and global max's. You can even use a number line to figure out where there are inflection points. I believe we use the second derivative to figure out inflection points. Besides the Line Test, I enjoy doing various problems like the classic optimizing problem. When I did a post on that I understood it more than if I were just to read it over. For me, making a post on work that I have learned helps me more. I also enjoy doing Antiderivatives. One thing that people might forget is to add (+) C. Remember to always add C! So test is tomorrow and I'm feel as if I need to review more of my notes because when I did the Practice Test, I didn't understand most of them. Though it’s a good thing we had group time to go through it, MR. K went over it in class and that Manny made a great post. It all helped!!!

Blogon Blog #5

Todays class was interesting! Pre-test today, yeaup.. scribe on it is down there. At first this unit was flowing like water to me, I got it all. I don't know happened... This pre-test was hard! At least for me. I got like 2 multiple choices right? And one part in the long answer? Ouch, it hurts. It might be because I haven't been doing alllll my homework, because I felt like it was enough. I guess not. I need to start cracking down full force on this calculus business. The optimization problems and related rate stuff are fun to me, because I know I can do it (most of them anyway). Most of my time was invested in those types of problems, but it wasn't on there. When I looked at the paper, I completely blanked as it looked completely new to me. It's an eye opener, cause the exam would probably be the same way! I think I just need to invest more time in more types of questions, and do allll of my homework, but that won't be till after the holidays (can't wait!). I just don't have anymore time. Well it's because I chose not to have any time by getting involved with things. Okay enough with my life story. I'm going to study study study for this test now, 3 hours already past just like that prior to doing my scribe earlier =(. GOODLUCK EVERYONE! And wish me luck , too... cause i need it =).

Scribe Post: Day 65

Well it's Manny and I'm your scribe for today! Todays class we did on our pre-test on our fifth unit on applications of derivatives. Here's the questions with the answer, and what I think the solutions are to get the answer.

Multiple Choice Part

1) The Graph of the function f(x)= 2x^5/3 - 5x^2/3 is increasing on which of the following intervals?I. 1 < x II. 0 < x < 1 III. x < 0The answer is I. and III. only.
- We get this by differentiating f into f'(x) = (10/3)x^2/3 - (10/3)x ^-1/3.
- Then we can factor it into f'(x) = (10/3)x ^-1/3(x-1) by taking out the lowest common factor to find its roots in order to make a number line
- We now know that there is a root at 0, and 1, so where ever it is positive, the function f is increasing.

2) Let f(x) = x⁵ - 3x² + 4. For how many inputs c between a=-2 and b=2 is it true that f(b) - f(a) / b - a = f'(c)
The answer is 2.
- First we'd find f(b) and f(a), which equals 24, and -40 respectively.
- Then plug it into the equation to get f'(c) to equal 16.
- Then differentiate f to get f'(x) = 5x⁴ - 6x.
- And since we're looking at the point f'(c), and we know what the point is, we get 16 = 5x⁴ - 6x.
- Then we'd just solve for the roots and we get the answer for how many we would have.
- I'd just punch in the equation the calculator and look at it over the [-2,2] interval and look at the zeros.

3) The table below gives some values of the derivative of a function g.


Based on this information it appears that on the interval covered by the table...
The answer is that g has a point of inflection.
- From the information, we know that g will be increasing everywhere, since it g' is positive throughout the interval.
- But we notice it g' starts to decrease after increasing, so at the highest point before decreasing there is a horizontal tangent line which means that there is a point of inflection because there is a change of sign from a positive to negative rate of change.

4) Suppose f is a continuous and differentiable function on the interval [0,1] and g(x) = f(3x). The table below gives some values of f

What is the approximate value of g'(0.1)?
The answer is approx. 3.84.
- For this we use the chain rule law.
- We know that g(x) = f(3x).
- So then we find g'(x) = f'(3x)(3) by the chain rule.
- After just plug in the values to get g'((.1)) = f'(3(.1))(3).
- Which turns into g'((.1)) = f'(.3)(3), then solve using the symmetric difference quotient.

5) If f(x) = ln(x) - k√(x) has a local minimum at x = 4 then the value of k is:
The answer is 1.
- If x = 4 is a minimum on the graph of f, then f'(4) = 0 must be true.
- So differiantiate f into f'(x) = 1/x - (1/2)k(x)^-1/2
- Then plug in the 4 f'(4) = 1/4 - (1/2)k(4)^-1/2
- Now solve for k.


Long Answer Part

1) Let f be a function given by f(x)= (3x - 2) / ( √(2x² + 1) )

a) Find the domain of f.
The answer is (-∞,∞)
When deciding domain, we need look only at the denominator. And in this function the denominator will never equal 0, meaning it's never undefined which means the function won't have vertical asymptotes. From that we know that the domain includes all the real numbers.

b) On the graph below, sketch the graph of f.


- We can sketch this by first looking where the function has roots. Roots occur when the function equals 0, or undefined. Look at both the numerator and denominator for roots. In this function the denominator can never equal 0 because of the square root function, which means there is no vertical asymptote. But in the numerator we notice that there is a root at 2/3.

- Then we can look at where we have y-intercepts, which occur when x=0. By plugging the 0 in for x into our function, it is easily located.

- Next, we can look at if theres any horizontal asymptote. And there is since the highest degree of power in the numerator is equal to the degree in the denominator. Horizontal asymptotes are discovered by the limits of infinity concepts. From this information we can just take the leading coefficient in the numerator which is 3, and divide it to the leading coefficient in the denominator which is √(2), so there is one at 3/√(2). And we can't forget about the -∞ side, to find that there is another horizontal asymptote at -3/√(2).

- Then we need to find out where the function is increasing and decreasing, and its concavity. For this we need the derivative. But the derivative was already given in part (d). So from finding the root to be -3/4, we make a number line to see that it is, decreasing to the left of it, and increasing to the right, which means there's a minimum at that point.

c) Write an equation for each horizontal asymptote of the graph of f.
The answer is lim x->∞ f(x)= 3/√(2) and lim x->-∞ f(x)= -3/√(2).
- Applying the infinity concept to our function. The -2 and 1, in the numerator and denominator respectively are insignificant to infinity, so we disregard it.
- So the denominator can be rewritten as √(2) * √(x²), which is √(2)*(x).

d) Find the range of f. [ Use f'(x) = 4x + 3 / (2x² + 1)^3/2 to justify your answer]
The answer is [ -17/4√(17/8), 3√(2) ).
- Since there's a minimum at x = -3/4, we can just plug in that value in our function to get the minimum output.
- Our maximum output is approaching 3/√(2) but never actually touching because of the asymptote there, found in part c).

Click here (part 1, part 2)to look at the paper with the correct circle answers and other multiple choices.

And that was it for the whole day. *Whew!* I'm a bit still unsure about parts of the long answer part, sooo if you're confused, I am too =). I tried my best to answer these problems in full detail as much to my understanding. Our group did pretty poorly on the test afterall. I'm scared for the test *gulp..* Anyway.. next scribe for Thursday will be Danny. Later days!

BOB

In this chapter, we have learned about the different applications of Derivatives. I really enjoyed this unit because I found it easy to understand. The only part that I had trouble with was the Optimization Problems. For me, it was very difficult to understand, especially the rate of travel. I have to clear this unit up before the test. I'll try to study hard on this part of the chapter. I hope everyone will do great on the test tomorrow!

BOB - Lindsay

This unit was a bit easier to understand than the previous units. Could it be that I'm getting better at this calculus business? I really do hope so. For the test, I think I should go over everything one more time because I usually miss SOMETHING. I'll somehow pack it all in this head of mine. I need some more practice on the optimization stuff and possibly antiderivatives but other than that, I think I'll be fine for the test. It's almost the winter break! Almost time to laze around and enjoy the luxury of doing nothing! I hope everyone does well on the upcoming test!

EDIT * nevermind I'm not ready. after that pre-test...boo it's 11:30 pm right now STUDY TIME.

BOB

MArk's BOB
I really enjoyed this unit. We did a lot of problem solving and group work for this unit. I found the problem solving the fun part in this unit. It was also fun to hear and learn with my fellow classmates. We had some disagreements and we had some clueless moments. We were there to learn as a group and we were there to help clarify anything that we found challenging. At this point in the course, i have realized that the way we think is different. At the beginning of the course, we were learning the basic idea about derivatives. Here we are now learning how to find anti-derivatives and we are learning how to solve optimization equations. In summary, i have not had much difficulty with this unit. I think it is understanding what steps to take to solve an equation/question. An example, solving word problems involving optimization.

Scribe Post: Day 64

Hi ya'll! This is Jann and I'll be your scribe for today. XD

Today, we continued our discussion on Antiderivatives. Here we go...

Last Friday, we started our discussion and we came up with the first antiderivative rule.
An antiderivative is basically just DIFFERENTIATING the function backwards. The rule for finding the FIRST derivative from the PARENT function has been reversed.

For example: Find the antiderivative of f'(x) = x.

Applying the rules for the antiderivative, we came up with the answer. If your wondering where the "C" came from, good for you! C is an unknown constant in the parent function. We don't have any evidences that a constant exists within the parent function.

Note: Remember that the derivative of any constant is 0.

What if...

For this example, we were given a point in the graph, (1,2). Using this information, we can identify the unknown constant, "C", by substituting the values into the parent function.
Then, we discussed the other antiderivative rules.Note: Remember that the derivative of cosX is -sinX. Therefore the "antiderivative" of "sinX" is the negative of "cosX".

Note: "Anything you can do, you can Undo..." (Mr.K) Every single derivative rule we have learned has an equivalent antiderivative rule!

How do we know if the antiderivative is correct? We can DIFFERENTIATE the antiderivative we came up with and see if the answer is the given question. It is the same as checking a set of factors by distributing the factors and see if the answer is the same as the given function.

For example:

f(x) = 2x^2 - 4x
= 2x(x-2)

Note: "Any high school level functions CAN be differentiated or antidifferentiated..." (Mr.K)

Then, we reviewed the "Definite Integral".
If you're wondering why antiderivatives have a stretched "s" like a definite integral, congratulations! The format of the antiderivative itself is formally known as an "Indefinite Integral". It's the opposite of a definite integral because it has NO limits/intervals (the number at the 2 ends of the "s").

Here are some examples we discussed:

If your wondering why number 3 has no answer, THERE IS NO ANSWER TO IT for now. No one knows the antiderivative for this function. If anyone answers this, that lucky person will be famous and his/her name will be written in the history books! Cool eh? XD

Lastly, we discussed this concept:
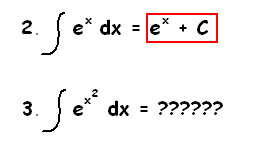
Correction: "no inflection points [f"(x) not equal 0]"

We explored the concept of this function. We found the first and second derivatives. Then, we used the first derivative to find the critical number, -b/2. Using this critical number, we can explore the graph of the function. Using three values, b>0, b=0, b<0,>

Whew... that took a while... XD. Anyways, I hope I covered all the things we did today.

Reminders: Pre-test tomorrow! Put up your BOB's before Wednesday.

Oh and BTW, Manny is scribe for tomorrow. XD

DAY 63

Heey guys! I know I said I'd have the post up by Friday, but I gave blood and fainted so I slept all day. Anyway, I'm good to go now, so let the calculus begin!
In order to solve these kind of problems, you'll need three things:
-the derivative
-the values of the closed interval plugged into the equation
-the Mean Value Theorem

First, we take the values in the closed interval, and plug those in to the equation.

Then, we find the Mean Value Theorem.

Next, we find the derivative of the function.

We take the Mean Value Theorem, and the derivative to solve for c.

Repeat

the same

steps

above to

solve for c.

2.

3.

HMWK is Exc. 5.6 Questions 1-14


Also, if you haven't already done so, comment on Christian's story http://pandaproject-apcalc06.blogspot.com/2006/11/pedro-pi-rate-panda-part-1.html for the Panda Project.


And, think about your integral pictures...


Last but not least, JANN is tomorrow's scribe =)

scribe

today we started class with a worksheet

topher and sully decided to go cut down a Christman tree together, so they ventured out into the snowy woods. after wandering for close to three hours, the two found the perfect tree but also decided that they were lost. luckily Sully found a map nailed to a tree which showed that they were four miles due north of a point perfectly straight road. six miles east of that point was the park that the two called home. if Topher and Sully can walk and drag the tree at the rate of two miles per hour through the snow, and three miles per hour on the road, what is the minimum amount of time that they would need to get home?








then we did examples on the board












homework 5.5
linger your next

Scribe Post

Hi! It's Katrin and I'm subbing in for Charlene...

Today we started off with 2 groupwork sheets based on optimization problems. The first sheet was pretty straight forward and I believe every group got it right. =)

Then came the second sheet!!! It was much more difficult than the first and wasn't as straight forward.

GROUP WORK SHEET #2: Willy's Pet Bob

2. Willy the Wallaby has been looking for the perfect pet for a very long time and he has finally found it - Bob the boa consticter. Now Willy has to make a closed, rectangular cage for Bob but it has to be 4000 cubic feet in volume and the length has to be 5 times that of the height of the cage. The material to make the cage costs $ 0.25 per square foot.

1) What are the dimensions of the least expensive cage?
2) How much does it cost?

.
























.

NOTE: Click on pic. and it will be easier to read.... =)

Then we went over the "CLASSIC OPTIMIZING QUESTION" that we didn't get to do yesterday...
.
You are standing at the edge of a slow moving river which is one mile wide and wish to return to your campground on the opposite side of the river. You can swim at 2mph and walk at 3 mph. You must first swim across the river to any point on the opposite side. From there you will walk to the campground which is one mile from the point directly across the river from where you start your swim. What route will take the least amount of time?

.









NOTE: THE RED BLOTCHY NUMBER IN THE NAVY BLUE BOX IS #3...












.
Homework: finish up 5.4 Excericises
.
The next scribe is Charlene ;]

Scribe post

Well, I guess I(Anh) am your scribe for today and I'd like to start off by thanking Lindsay for taking my scribe day yesterday =). Anyways we didn't get much done in class today because of the fire bell...thats $500.00 out of the school's funds for something that I don't think was necessary. It was a lot of fun freezing in the cold outside though (trying to be sarcastic)...haha..anyways onto the math.

We started the class of with questions on the board like always...

question 1

An open rectangular box with a square base is made from 48 ft squared of material. What dimensions will result in a box with largest possible volume?


question 2

A sheet of cardboard 3 ft by 4 ft will ne amde into a box by cutting equal sized squares from each corner and folding the four edges. What will be the dimensions of the box with the largest volume?


question 3

A cylindrical can is to hold 20π m cubed. The material for the toop and bottom costs $10/m squared and the material for the sides cost $8/m squared. Find the radius, r, and height, h, of the most economical can.



Mr.K was trying to be ambitous like he always is and tried really hard to squeeze in the "classic" optimizing question but we were saved by the bell.


Homework is ex 5.4 questions 10-18
Test is on Friday! So study!!!

Anyways I was so hungry I decided to draw this..=)


Next scribe : Charlene =)

Scribe Post

This is Lindsay subbing for Anh!! =D

To start things off, I would like to remind you guys that Mr.K gave us an extension for our derivative photos. You have until the end of the week to make it great.

Next, the white board questions! I like to draw weird cartoon things. If find it easier to read things when there's something drawing your attention to it. So, I drew. XD. I also use sticky notes...and I don't like typing much either -_-;; I prefer paper and pen...yes, anyway, moving on..

1. Evaluate these limits:

2. Determine the total number of max and min points of the function whose derivative is:

3. Build a rectangular pen with 3 parallel partitions using 500 feet of fencing. What dimensions will maximize the area of the pen?

4. An open rectangular box with square base is made from 48 ft^2 of material. What dimensinos will result in a box with largest possible volume?

CONSTRAINT: 48 ft^2, surface area
OPTIMIZE: volume

*NOTE: We'll work on this problem tomorrow!!


5. Find the point (x,y) on the graph f(x)=root of x closest to the point (4,o)




















Next scribe is ANH!!!!

homework: 5.4 1-9

Scribe Post

Hi guys, I will be your waiter… I mean... scribe for today :)

We started off today’s class by going over Friday’s Quiz. I think the first question was quite straightforward so I won’t get into that.

2) Use linear approximation to find the value of (1.001)^100. I had trouble understanding this question, but after Mr.K explained it, it made much more sense. The important thing to recognixe is that you have a value very close to one that would be easy to solve for.






1) Find a function to represent the question. We also find the derivative of the function, so that we can find the equation of the line later.

2) Choose a simple number close to the value we’re approximating. In this case, 1 is the easiest value. By finding the f(1) and f ‘(1), we can use the point-slope formula to find the tangent line.

3)Now we can plug in the value we’re approximating, 1.001, and we get an answer of 1.1, which is a good approximation of the actual value, 1.1051.


3) Using 3 iterations of Newton’s Method, by hand, find the root of f(x)= x^5-x+1.
Start with x= -1.




Newton’s method uses progressively more accurate tangent lines to approximate the root of a function. First of all we need to have f(x) and f ‘(x) so that we can find the tangent line.

The second step shows the familiar point-slope formula. Because the root is where y = 0, we can get rid of y, and then rearrange the equation to solve for the next x-value.

The first iteration is done by finding f(-1) and f ‘(-1). Then plugging these into the rearranged formula, we have the next x-value. We repeat the process using this next value instead of x=-1, and so on until reaching however accurate a solution we want.


From here we moved on to the lesson itself, all about finding horizontal and vertical asymptotes and using derivatives to find out what's happening around the asymptotes.

1) Draw a graph of f(x)= 1/(x-3)




Because the limit of the function as x approaches 3 is one divided by zero (undefined), we know that there is a vertical asymptote at x=3. If the limit were zero divided by zero, it would be indeterminate (impossible to know what is happening).

By finding where the function is increasing/decreasing and concave up/down, we can determine what the graph is doing on either side of the asymptote. We know that there are no roots because the numerator always exists. So we get a graph like this:


2) Draw a graph of g(x)= 1/(x^2+1)

Once again, the numerator always exists so there are no roots. And there are no vertical asymptotes because the denominator will always exist. By finding the limit as x approaches infinity, we can determine that there is a horizontal asymptote at y=0.
Technically, we should also find the limit as x approaches negative infinity, in case the graph is doing something else on the other side of the x-axis, but this is a nice happy function so we don’t bother.



From here it’s more or less the same as the previous question. Finding g’ and g”, we learn key information about the graph that allows us to draw it. For example, we know there’s a max at (0,1) because g’ changes from positive to negative at x+0.



This graph is a very well-known one in mathmatics, called the 'Witch of Agnesi". To find out why, look here: http://mathworld.wolfram.com/WitchofAgnesi.html

3) Next we looked at (2x^2)/(x^2-5x+6).

If we try to find the limit as x approaches infinity, we will get ∞/∞, which is indeterminate. In this case, to find the horizontal asymptote we divide each term by the highest power of x in the function. There are three possible outcomes when we do this:

1) If the degree of the numerator is less than the degree of the denominator, the horizontal asymptote is at y=0.
2) If the degree of the numerator is greater than the degree of the denominator, the horizontal asymptote does not exist.
3) If the degree of the numerator is equal to the degree of the denominator, the horizontal asymptote is the leading coefficient of the numerator divided by the leading coefficient of the denominator.

It is probably easier to memorize these three rules, but if you think you will forget them over time, it is probably better to remember that dividing by the highest power of x will allow you to find the horizontal asymptote. That's what Sherlock Holmes would do, anyway. :)



In this problem, the third case applies. So we get an asymptote at 2/1.
If the numerator had been 2x^3, the second case would apply and the horizontal asymptote wouldn’t exist.
And if the x^2 in the denominator had been x^3, then the horizontal asymptote would have been at y=0.

So that’s about it. Just remember...

By finding the limit as x approaches infinity you can find any horizontal asymptotes.

If the limit as x approaches some value a approaches infinity, there's a vertical asymptote.

And Jupiter has 63 known moons.

Next scribe shall be… Anh.