We discussed how the pre-test went with the substitute and the multiple choice questions were pretty straight forward and no one really had any problems. What did leave us all puzzled was the long question in the back, the "open response".
The question was . . .
(1) Let f be a differentiable function such that f " is continuous and f and f ' have the values given in the table below.
(now I wished I had the smart board so I could draw the box like Mr. K had done in class..ah the wonders of technology, I honestly can't keep up with it.)

Don't forget the mnemonic " LIATE ".
L ogarithmic
I nverse trignometric
A lgebraic
T rignometric
E xponential
So an example problem that we did that even got Mr. K, who's a math gyro (uh I don't know if I am using that word right) thinking was . . .
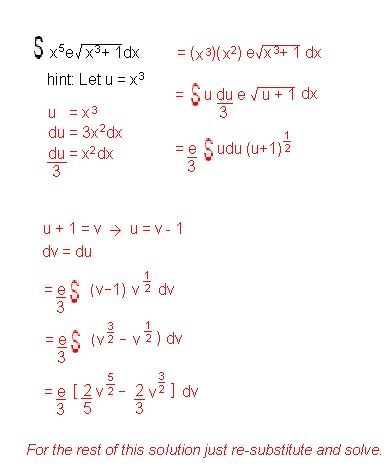
So to review . . .
+ When the integral is a composite of functions, you are better off using substitution.
+ when the integral is a product of functions, you are better off using integration by parts.
Last but not least next scribe is Suzanne.

No comments:
Post a Comment