SCRIBE POST DAY 34: Calculating Areas; Riemann Sums

Hey guys, HAPPY HALLOWEEN =) Just a note to Suzanne, and Jann that your Scribe Posts were day 33 and 32, because Charlene's was 31. Anyway, let's begin... today, there was only one question on the board

The velocity of an object, in feet per second, is given in the table.

a) Sketch a velocity-time graph from the table.

b) Give lower and upper estimates for distance traveled in the 5 seconds.

c) Estimate actual distance traveled.

d) Show a representation of the lower estimate in terms of a shaded region on the graph.

b) LOWER ESTIMATE:

(1.4)(1) + (2.7)(1) + (3.5)(1) + (4.5)(1) + (5)(1)
= 17.1 ft.

UPPER ESTIMATE:
(2.7)(1) + (3.5)(1) + (4.5)(1) + (5)(1) + (5.7)(1)
= 21.4 ft.

c) [17.1+ 21.4] / 2
= 19.25 ft.

d) The graph is monotonic increasing, so the lower estimate can be found by the left hand sum. This part is shaded in grey.

We then looked at different examples and distinguished the difference between integrals and areas.

Area is width multiplied by the height. Integral is the signed area under a curve. If you look at the first example, from 0 to 3 the area you would get is 9/2. You would also get the same area from -3 to 0. But if we're talking about integrals, the integral from 0 to 3 is 9/2 and the integral from -3 to 0 is -9/2.

Notice that in the first and second examples, we're easily able to find the area because the functions are shaped nicely... into triangles and a half circle. In the third example however, we get a parabola... so to find the area, we'd have to find the different values at different intervals and add the values together as we've done on our previous questions.

After this, we programmed Riemann's sum (RSUM) into our calculators. As usual, we ran out of time soo if you guys missed the steps, just turn to your handy dandy textbooks and you'll find it all on page 168.

The HOMEWORK for tonight is Excercise 3.2 all ODD questions only. And tomorrow's scribe shall be MARK.

Won't let you fall...

In today's class, we began our third unit. So far, so good. I guess the reason why I'm here is about the whole procrastination thing. I understand where Christian is coming from when he says that he wants to have a conversation with it. I mean, we have this BLOG here for a reason... and I think that we're not using it to our advantage. I know everyone has a million other things to do, but it seems like nobody reads the blog anymore... except to scroll down at the bottom just to see if you're the next scribe. In the beginning of the year, we'd comment each other on our posts. If you guys were to go back to the most recent ones, nobody has left comments. I mean how are we going to be in the HALL OF FAME if nobody reads the blog? I'm kind of disappointed, because I know that when it's my turn to post a scribe, I put a lot of effort into it... don't you guys do the same? Doesn't it feel disappointing when nobody leaves a comment? It makes me think that nobody learned anything from my post, and that I did all that work for nothing. I think that relates to what Christian was trying to get at. Mr. K always says that "MATH IS A CONVERSATION". My question to all of you is, why isn't the conversation happening? This is a whole new unit; we can still help each other out. Mr. K also said in today's class that we need to find ways to catch each other's falls... I am VERY willing to catch your fall, I wonder if anyone is willing to catch mine.

Linger =)

Scribe Post: Day 33

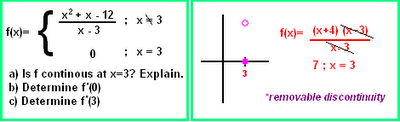
Well, I was completely surprised to find out that I was scribe for today. This being due to the fact that my internet was being evil this weekend, and I didn't realize we were already on round 3 of the scribe (this year's passing by so fast!) So this post is brought to you by Linger, who informed me of my duty (thanks again!)

Today we got started on chapter 3. This is the problem we looked at in class:


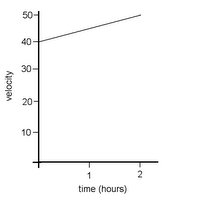
Manny is driving through the USA to Fargo. Between noon and 2pm his speed increases steadily from 40mph to 5mph.

1)Draw a graph of his speed over time.
2)Estimate his minimum distance travelled.
3)Estimate his maximum distance travelled.

1)





2) d=vt 3) d=vt
=(40)(2) =(50)(2)
=80 miles =100 miles

So, how did we get the answers for questions 2 and 3?

First, we are looking for the minimum possible distance travelled over the entire 2 hour period. We know that he was travelling at at least 40 mph throughout the entire trip. So by using the formula d=vt, (which most people probably know from physics) we get our answer. We know that there is no way that Manny could have travelled less than 80 miles. But because we know that he travelled at more than 40 mph as the trip went on, we know that 80 miles is an underestimate.

The same applies to question 3. We know that Manny was never travelling more than 50 mph throughout the trip. So he can't possibly have travelled more than 100 miles in that time. And because we know that it was only at the end that he reached a speed of 50mph, we know that 100 miles is an overestimate.

So now we know that the distance travelled over 2 hours is somewhere between 80 and 100 miles. We can also look at the solution to these questions from a graphical perspective.


2) 3)








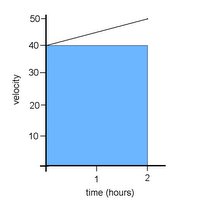
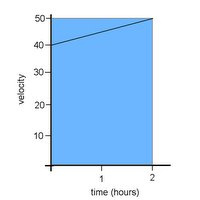
In grade 10 science most of us learned that we could find distance on a velocity-time graph by finding the area underneath the line. This is exactly what we did to solve for questions 2 and 3, except that now we are no longer dealing with nice, happy horizontal lines. So the minimum and maximum possible values we found earlier don't show us the exact distance travelled, because we are not considering the rate of change in our answers. Instead, we're saying:

If the velocity had been 40mph over the entire trip then the distance would be 80 miles.
If the velocity had been 50mph over the entire trip then the distance would be 100 miles.

This only gives us a rough estimate of the actual distance travelled. The area is either underestimated or overestimated, our margin of error is shown by the white triangle under the first graph, and the blue one over the second graph.
So, the next step is: how do we make our answer more accurate? What if we use the same method as before, but look at each hour seperately? We get these graphs:





2) 3)


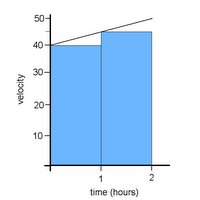
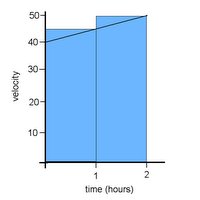
And by calculating the total shaded areas and adding them together, we find:

2) d1= (40)(1) d2= (45)(1) 3) d1= (45)(1) d2= (50)(1)
total d= 85 miles total d= 95 miles

Before we knew that the total distance travelled was between 80 and 100 miles.
Now we know that the total distance travelled is between 85 and 95 miles.
So we have narrowed down our error by half, from 20 miles to just 10.
We can also see the margin of error decreasing from the graphical perspective, as we see the triangles showing underestimation or overestimation are decreasing in area.
And if I were to feel up to making even more graphs, with more lovely blue rectangles, an even more accurate answer could be found.

Now, imaginate that we have an infinite number of little blue rectangles on our graph. The margin of error approaches zero, and we have an accurate answer, which makes us very happy :) In other words, we're just finding the exact area underneath the line.

Now, for a bit of technical stuff. How do we know if we are underestimating or overestimating?

When the graph is increasing, like the one in our example today:
If we take the areas using velocities starting from the left, the answer will be an underestimate.
If we take the areas using velocities starting from the right, the answer will be an overestimate.

When the graph is decreasing:
If we take the areas using velocities starting from the left, the answer will be an overestimate.
If we take the areas using velocities starting from the right, the answer will be an underestimate.

And the new term of the day is Monotonic. This means that the function is always increasing or always decreasing.

Tomorrow's scribe is Linger. Happy Haloween tomorrow, everyone.
And I can't get this to look quite the way I want, so I'm sorry if there are weird unecessary spaces or the numbers get squashed. I don't know how to fix it.

Bob # 3 B

Hello guys!

I'm encouraged by Lani's post, and her comment on my Bob # 3 to continue the dialogue about procrastination. I guess we had to cram and do our Bobs before the test, so instead of having a constructive conversation, we just put what we thought on our bobs. Well, to organize things, and to get the dialogue going, if anyone has a comment on procrastination, may s/he please do it here?

As for me, here's my PROGRESS REPORT since that day I wrote my bob:

Well, believe it or not, most of my homework was done by the morning of Saturday =). It's pretty interesting, because I was convinced before that procrastination works for me, and I should keep doing it. Well, what REALLY works for me is a whole afternoon free, with nothing to worry about, sipping my coffee (it's not good i'm really getting addicted) and reading my book. THAT is what works for me. As well, since then, I thought more about "knowing yourself", and I asked myself, "do you really know yourself well enough to call for people to do that?", and came to the conclusion that I don't. It's an ongoing process don't you think? Well, I'll make an attempt to stop being a procrastinator. I won't make any promises; I'll just do my best. That's all for today =)

BOB # 3

GEEZ PEOPLE!!! READ MY BOB # 2 AND VISIT THIS BLOG MORE OFTEN!!! I WAS EXPECTING 10 COMMENTS, BUT I'VE HAD ZIP!!! PLEASE VISIT MY BOB # 2 >='{


Anyhow, that's my ranting for today. I'm bored, so I thought, why not do the BOB now and not worry about it anymore? So here it goes.

Resources. This blog will be about the definition, purpose, and use of a resource.

Webster's defines a resource as a source of aid or support that may be drawn upon when needed. We often say in our bobs or comments that we don't understand a certain question, topic and so on. That's valid because we're not Einstein's (yet), but as responsible students, we are supposed to do all we can to find the answers to our questions. We can't just sit and wait; we have to scrutinize our notes, maybe ask our teachers or parents, go to our blog, read the book and so on. These are just some of the resources available to us. The concern is, we aren't making use of these, isn't that right? Mr. K, in the beginning of the year, said that there was a student who claimed, "Mr. K, I don't know what to do. I'm failing your class, although I listen intently to your lectures...", or something to this effect. "Well," Mr. K said, "have you been doing your exercises? Have you formed a study group? Have you tried to ask me for clarification on some of the questions you have?". To all of these, the student said no. This student only has himself to blame for his failure, because all the help he could have gotten was around him, but he did not make use of them.

Well, it's the same for us. Point is, WE HAVE MR. K, WE HAVE A BOOK, WE HAVE THE INTERNET, AND WE HAVE A (FRIGGIN) BLOG! Let's take advantage of all of these. I must keep this in mind, as much as all of you do.

oh oh and... READ MY BLOG # 2 AND COMMENT =)

“Project Emancipation Procrastination”

I’ve read and reread your posts on Ashlynn’s “Project Emancipation Procrastination” with great admiration and interest. Each of you has honestly laid out your thoughts and feelings on procrastination; I find it pretty incredible that you wrestle with this publicly; in other words, I find it to be more than “awesome” as it can be a toughie for anyone. For as Ashlynn notes:

Even the best and most gifted people can be procrastinators! You may even be reading this right now =P Even the incredibly talented Leonardo da Vinci was a procrastinator.

And many of you responded with your stories of procrastination so we know we are all in this together:

There are things I need to work out with myself, tendencies that I shouldn't have to begin with. I really don't know how they developed, but I have them and they're a problem for me. JessicaJill

Procrastination more or less starts off as a bad habit. Once you get into the habit of pushing things aside and waiting for the last minute to get it done that's when it becomes procrastination. It can take only one time for someone to get into this habit and it can become very very hard to get out. This is the case I'm in. Danny

Procrastination, in my opinion, is a habit, more than a disease. It is when one does things later, rather than sooner. Is it bad? Maybe. But what if that works for the person? Christian

My motivation to procrastinate falls under everything that causes anyone to procrastinate. I know it's sad, but I would rather go cloud watching and do nothing anytime! (I hope that comment doesn't affect my marks =P). But things have to be done =]. Manny

And some of you shared some suggestions:

Procrastination.....something that I'm sadly a part of. BUT then again....Who doesn't procrastinate and who hasn't procrastinated? I believe that everyone in their lifetime has had procrastinated at least once in their life spand. --- To avoid/solve procrastination, I should just finish whatever I'm going to do, right when I get it in the first place. Katrin

The trick I use in avoiding it... I tell myself that if I get my homework done, I can watch my tv shows or go to sleep early. It works for me, because I hardly have free time. All I've been doing is going to school, work, doing homework, signed up for a million committees, eating and sleeping. There's always something to do, so I know that putting one thing off no matter how easy it may be to catch up on, will put me behind.. and I for one can not afford that. Grade 12 is the year that counts Linger

What can I do to avoid it? I guess I should stay away from my room when I get home because it's the most distracting place for me. I should just do my homework before going into my room. Or do homework when I wake up (I wake up very early). I have about three hours to do what I have to do before school starts. Hopefully, I'll follow my own advice. Lindsay

So solutions to procrastination? Well, first you can get an agenda and plan your afternoon. Try to relax when you get home and eat. Mr.K said, "Set a time for yourself to do homework and do that everyday, to get your body used to doing homework." You can also try playing an instrument or playing your favorite sport. Your mind will get the stress off your head and you will get the blood flowing. Lastly, i think taking a hot shower can help, lol. The blood flows and loosens the clots in the brain, making you more relaxed. Mark

I think it is really quite unrealistic for me to try to stop procrastinating, so this is an honest way of improving myself and getting things done. If any of you think that making a schedule or locking yourself in an empty room after school with no way out is a bit too extreme, maybe this method can help you get things in a bit more control. Suzanne

Suzanne suggested an article that might be of value; one she saw helped her :

Here's the article: http://www.structuredprocrastination.com/
And thanks to the author, John Perry, for inspiring me to trick myself into working :)

In your thoughts on procrastination, the word "habit" was mentioned more than once and some of your solutions seemed to me to refer back to the same. I am wondering if indeed that might be a direction for the discussion to continue? Do you think? And in my wondering, I posted without a meaningful preface the following poem:

I am your constant companion. I am your greatest helper, or your heaviest burden. I will push you onward, or drag you down to failure. I am at your command. Half of the task you do, you may just as well turn over to me. I will do them quickly and correctly.

I am easily managed, but you must be firm with me. Show me exactly how you want something done. After a few lessons I will do it automatically. I am the servant of all great people; of all great failures as well. Those who are great, I have made great. Those who are failures, I have made failures.

I am not a machine, though I work with all the precision of a machine, plus the intelligence of a person. You may run me for profit, or run me for ruin--it makes no difference to me.

Take me -- train me -- be firm with me, and I will place the world at your feet. Be easy with me, and I will destroy you!

Who Am I ?

Do you find this true for you? How can we help each become emanicipated from procrastination? Can compiling ideas from the proposed solutions help? And/or can looking at "habits" that lead to less procrastination be of value?

And by the way, I'm not procrastinating now because I consider this discussion one of my "rocks". More on that later---

BOB #2

It's time for another test. I know I'm doing this at a late hour, but I wanted to get it done. I haven't been attending class regularly, nor have I been doing my homework, which will all show on the test. But, despite my bad habits, I'm still going to try and go to class. I have to be straight forward and say that I'm a horrible student, and me not coming to class is just me demonstrating how lazy I am. It's nothing to be proud of. There are things I need to work out with myself, tendencies that I shouldn't have to begin with. I really don't know how they developed, but I have them and they're a problem for me.

This however, has nothing to do with Mr. K's teaching methods. Mr. K is a great teacher, and instead of taking a grasp at this knowledge he's trying to pass on, I take it for granted. I seem to be pushing aside my own knowledge as well.

I think to myself how, for the last couple of years, school seems more like a chore to me, instead of something I'd want to do. I know that growing up I was always excited to learn new things, and take it upon myself to do some independent research on my own; not for marks, but for self gratification in knowing that I can attain all the knowledge that I can.

I assume that my interest in learning was never encouraged enough as a child, but I don't think I should try and find excuses for my behaviour. I suppose I'm just rambling. My point is, that I'm far from prepared, but I'm willing to face the consequences that I only brought upon myself. I know I'll feel ashamed and embarassed when I can't fill out the questions or I'm done before everyone because I won't know the answers. I did this to me, no one else, and I'd rather have you all know this now, instead of shaking your heads tomorrow. This test is not only questioning my intellect, but as well as my integrity.

Good luck to everyone else on the test.

BOB #2

Procrastination more or less starts off as a bad habit. Once you get into the habit of pushing things aside and waiting for the last minute to get it done that's when it becomes procrastination. It can take only one time for someone to get into this habit and it can become very very hard to get out. This is the case I'm in. Procrastination played a big part in why I got mid 70's and mid 80's in my classes throughout high school instead of high 80's and 90's. Every time I put things aside I get this small little guilty feeling inside of me and I'd eventually give in a do whatever it was I had to do. But this has not been the case for the year of 2006. I still get that guilty feeling inside of me but I don't always give in to it anymore. I don't need to explain why because I've already said so much in my first BOB =S

I'm going to go completely off topic just because I was thinking of this with my coworker the other day and I feel that I should share it with all you guys. We were talking about how the new guy at our work started with an hourly salary of $8 when I was only making $7.75 after working there for a little over a year. We both felt I deserved a raise to at least $8 and she told me to tell the boss to give me one. That's when I remembered what my dad had told me. He said never ask someone for this or that if you think you deserve it because if you do then you probably don't deserve it. If you think you deserve something then it'll be noticed by whoever without even having you mention it. Why you say? Because you deserved it. He always believed that actions speak louder then words and if people noticed, you wouldn't have to say anything to get what you deserve. So just for the heck of hearing your guys opinions on this, what do you think? If you think you deserve something would you let it be known? Should it be known? Has this ever happened to you before? What did you do in that situation? After reading my ramblings do you think differently about it? You don't have to comment on this, it's just something I'm putting up because I feel it may help not just in class but life in general.

BOB #1

That's right, the night before our SECOND TEST and I'm posting my FIRST BOB. I think this is totally way over procrastination for me, and I'm extremely disappointed in myself for this long long wait for my BOB. I'd first like to start off with an apology to Mr. K for having this up so late.

Basically I want to reflect on how I managed through the first unit of calculus. I'm going to be completely honest and I'm not going to "sugar coat" any of the things I feel about my working ability in this class. Since this is my upgrading year I figured things would be a little more relaxed and light for me, however that wasn't the case. I'd say I started off strong in this class and after week one everything went downward from there. I've never been able to finish any of my exercises, sometimes even missing a complete exercise in all. However, and this is not a lie, I pay very close attention in class and understand things that are being done. I find it a lot easier to work and understand topics in groups with interaction and conversations, rather then doing things in silence and independently. I know tests won't ever be done in groups so I have to find some way to make it easier for me in that regard.

When it comes to this class, it's not that I don't want to learn it's just that I never realized how horrible I am at tackling multiple things in life. I'm currently taking this course, chemistry, and I have choir last period. Every Mondays, Wednesdays, and Thursdays I have Vocal Jazz practice after school. Six out of seven days of the week I'm working evenings or during the day if it's Saturday and Sunday. That leaves me with the night hours after 10 pm every evening to do the homework for this course. The spares I have between chemistry and choir are used to do chemistry homework or resting because I'm always so tired from working evenings and waking up early for school. They're mainly used for resting however because there's not a ton of chemistry homework. This has been the reason or some would say excuse for me not being "able" to finish my homework in this class. I'm a very sensitive an emotional person and there's been things in my "high school life" that's had me go off track numerous times. I'm trying to set things right and get back to being the student I know I can be. It's taking me longer then I had hoped but either way I got to find a way to get it done this year. I didn't stay in high school because of my marks, I stayed because of my attitude toward learning, toward education wasn't positive anymore. I couldn't see myself going into post secondary and wasting my money knowing I wasn't being myself in school. So I chose to stay, to try and right my ship and possibly get things done.

BOB # 2

A CHALLENGE TO EVERYONE! And Please Read the Whole Thing or I’ll be Sad >=’[

I’ll try to do two things for my this, my second bob. The first (1) is that I’ll strive to be honest in this post. I won’t care about image and formalities; I’ll just be frank. My second (2) goal for this bob is to get a message across to all of you. I’ll bug and bug and bug bug bug the class until I get a comment and have a dialogue about this. Procrastination. Yes, almost all of us did a bob on this, but this would be different. TRUST ME.

I'm intrigued by this topic because it affects the lives of many people in crucial ways. In the beginning of the year, Mr. K said the scribe posts should be posted no later than 9:00 PM, right? But why do we all post later than that? Well, we all know the answer to that. Procrastination, in my opinion, is a habit, more than a disease. It is when one does things later, rather than sooner. Is it bad? Maybe. But what if that works for the person?

Some people know that I’m a bit busy (same with everyone else), especially this school year. Some students ask where I get the time to do all the things I do. Yet, despite this, I can probably claim that I’m the High King of Procrastination! Suzanne, Linger and Lindsay can confirm this. I don’t do things until the very last second. The reason for this, I tell my friends and parents, is because I can’t do homework if I’m feeling crappy! So, I watch the TV first, I surf the internet, chat on msn, eat food, read a book, possibly nap. In between, I tend to my duties at school, home and other places; e.g. preparing things for an event at my church, doing student council stuff through e-mail (contacting people, designating duties blah blah blah).

It’s probably not good, but right now, homework isn’t on the top of my list. This doesn’t mean I don’t give regard to my education; in fact, it’s the opposite. Over the past year, there’s been tumult in my life, reason enough I think for someone to neglect his education and all else. However, I still go to school religiously, doing most that I can do, right? Point is, I tried to find out who I really am, countless times, and found out what works for me and what doesn’t. It just so happens that what works for me is I need to feel lighthearted first, before I tackle tedious stuff (such as calculus homework.. SORRY MR. K!). I just won’t be able to stand, after walking for an hour going home + a million meetings + a million arguments, to do homework right away. Gosh, if I do that I might get a stroke.

This is my challenge for all of you. By the end of this year, find out what works for you and not. Be HONEST TO YOURSELF. Be it ways of tackling homework, or food preference, type of people, whatever.. find what works for you, and stick to it! It’ll benefit you in high school and beyond. As for me, I’d still be here, eating my oreo and caramel mcflurry, watching tv *cough*calculuslater*cough*. It’s all about responsibility. My responsibility is to do the things I committed on doing, when I say they’d be done, to the best of my abilities, period. That’s my little confession for today.

BOB #2

Well this is our first step into AP Calculus. It is about Derivitive Functions. Now we have a test tomorrow on it. This Chapter was actually very difficult for me in the beginning. I was really lost in class. However I studied a lot and asked my peers questions, so that really helped me. Procrastination is also one of my big negatives. I was behind on my homework and it was really hard to catch up, especially if the topic was discussed days ago. Now I'm going to do my homework on the day that it is assigned. I will remember our discussions in class so it will be easier. To answer Ashlynn's question I think the reason for procrastination is because we always tell ourselves that we'll get it done and we believe it; the case is always the same, it never gets done. Procrastination can lead to laziness and just giving up because it's "too late" to solve. Remember the Forgetting Curve! We can all avoid this.

What really helped me was all the reviews that we did. We may be a little bit behind, but at least we're confident in what we learned. I liked how if we asked for a review on anything then we would do it right there on one or more questions, even if it was in the middle of another discussion. Those spontaneous reviews were extremely effective.

Also, I liked how we seperated into groups. I usually work well individually, but in Calculus I prefer working in groups. I learn way better. Three brains is better then one! This is how I figured out to solve my mistakes. Things were more clearer especially after when we did an overall check of the questions.

BOB NUMBER 2

Like always, today's class just passed me by... and I feel like time's just going by so quickly because it means that we're getting closer and closer to writing that test tomorrow. I was confused about things, but after that day of review in class, I felt so much more at ease and when Mr. K was talking, I was like "OMG, I get what he's talking about now!!" lol, I felt so happy XD

To answer Ashlynn's blog prompt... I think the main reason for procrastination is knowing ahead how much time you have to get something done. For example, many of us get school projects. The teacher usually lets you know that it isn't due for another two weeks. So you get home and you end up putting it off, because in the back of your head you're thinking... I'll do it tomorrow. Sooner or later, tomorrow comes and there you are... empty-handed or unsatisfied with your work. It can affect us all, because if we make this a habit, it'll be stuck with us for the rest of our lives.

The trick I use in avoiding it... I tell myself that if I get my homework done, I can watch my tv shows or go to sleep early. It works for me, because I hardly have free time. All I've been doing is going to school, work, doing homework, signed up for a million committees, eating and sleeping. There's always something to do, so I know that putting one thing off no matter how easy it may be to catch up on, will put me behind.. and I for one can not afford that. Grade 12 is the year that counts guys.... so don't let procrastination hold YOU back from graduating.

Speaking of PROCRASTINATION, I really SHOULD be studying.... good luck on your tests =)

Scribe Post: Day 32

Hi ya'll! This is JANN and I'll be your scribe for today.

Before going over what we did today, I just want to remind everyone about the test tomorrow. I hope everyone will do great! XD Anyways, here we go:

In the beginning of class, we went over the last 2 questions in the pre-test.

(5) Suppose a function "f" is defined so that it has derivatives:

Over what interval is the graph of "f" both increasing and concave up?

Solution:

To identify what interval is "f" increasing and concave up, first find the roots of the two equations:

f'(x)= x^2(x-1)= (x^2+2)(x-1), x= 0,1
f"(x)= x(3x-2)= (x+0)(3x-2), x= 0,2/3

Then, use the number line method for each equation.



We can see that at the intervals x>1 and x>2/3, the answer is positive. Therefore, the graph is increasing due to f' and concave up due to f" since both are positive.
The answer is x>1. The number 1 is not included because the graph increases after x=1.

(6) Consider the following table of data for the function "f".



(a) Estimate f'(5.2) as acurately as possible.

Solution:

In order to estimate f'(5.2), we use the Symmetric Difference Quotient method. Since the given is a table of values, we can get the slope to the left and to the right of x= 5.2 Then, we get the average of the 2 slopes.



(b) Write the equation for the tangent line at x= 5.2

Solution:

To get the equation of the tangent line at x= 5.2, we could use the point-slope formula using the pt (5.2,8.8) and the answer to 6a.

y-y1= m(x-x1)
y-8.8= -9/4(x-5.2)

(c) Use your answer to part b to find approximately, f(5.26)

Solution:

Using the formula found in part b, we could simply substitute f(5.26) as "y" and 5.56 as "x".

y-8.8= -9/4(x-5.2)
f(5.26)-8.8= -9/4(5.26-5.2)

(d) What is the sign of f"(5.2)? Explain your answer.

Solution:

f"(5.2) is "negative" because the first derivative of the parent function is decreasing due to the slopes -2 and -2.25 Because the slopes are decreasing, the 2nd derivative at these points are negative.

After going over the last 2 questions, we were given another group problem. Here's the function of the problem.



I forgot how the questions were typed, so here are the answers anyways. XD

Solution: The graph of the function



The graph is constantly increasing from negative infinity up to x=3. As soon as it reaches x>3, the graph jumps up in a parabolic funcion. Therefore the graph is discontinuous and nonremovable. Its not a good idea to but grain from this company because if you buy more than 3 bags at $9, the next bags would be higher that the rate of the first 3 bags.

Finally, we were given 2 problems.

1)

a) Is "f" continuous at x=3? NO
b) Determine f'(0)= 1
c) Determine f'(3)= does not exist

2)

a) On what interval is the graph of "f" concave down? (-3,-2)
b) Where on the graph of "f" will there be a point of inflection? x=-2
c) If "f" is decreasing at x= -2.5, is "f" increasing or decreasing at x=-2.Explain Decreasing

Tip of the Day: Enter nDeriv(Y1,X,X) in your Y0 and leave it there until we throw away our calculator, I guess. =P If you put this on your calc, you dont have to re-enter this command in Y2 every time you need to find the derivative of the function in Y1.

I think thats all we did today. Sorry if my post is colorless(besides blue and black). I dont know why, but the tool bar on top is pretty... short? The color and font size menus are missing. 0.0 Anyways, good luck to ya'll tomorrow!! =D

Oh yah I almost forgot. Next Scribe for Monday is... Suzanne!

BOB 2

Ok, here's my 2nd BOB. Today, we did our pre-test. It really helped me understand the concepts more in this unit. In Chapter 2, I didnt quite had any problems unlike the first chapter. I had trouble with the first one because it was a review from grade 12. So far, I understand the concepts of a derivative. Last Tuesday, Mr. K discussed the things that we had trouble with. It was really helpful because I understood the unit more. =)

BOB #2

Well I just finished posting up my 1st BOB a few minutes ago. That was my plan for like ages. Here's my sencond... ;]

Like I said in my first BOB, all we did today was worked on a pre-test on the derivative function. I wasn't sure about some of my answers but when i worked with my group, they showed me how to derive them. I found that distinguishing between the parent function, derivative fuction and the 2nd derivative function was a bit confusing and hard because of the different rules. Later in the day, when I had help from Mr.K, I had a better understanding of those rules because I listened to every detail Mr. K was saying. Having a better understanding of the rules, sparked that "moment of clarity" for me and I felt happy =) and a sense of relief that i had an understanding of that material. In class, I get distracted by writing notes about whatever Mr. K is writing and talking about. I learned that it's better to listen because that way the material that Mr.K is teaching just doesn't go in one ear and out the other.

Ashlynn's Blog Prompt:

Procrastination.....something that I'm sadly a part of. BUT then again....Who doesn't procrastinate and who hasn't procrastinated? I believe that everyone in their lifetime has had procrastinated at least once in their life spand. I also believe that the causes of procrastination may be [1]a person is too tired from school to do anything, [2]too busy earning a living by working, [3]afterschool activities get in the way, [4]interent/computer/tv distractions, [infinity] etc., etc..... Procrastination can affect anyone at anytime whether it is at school, home or anywhere else. At school, I procrastinate especially during second period spare. We usually are to busy eating and chatting about whatever, to even do any homework. Like for instance, today, we were going to do our Physics review sheet but we ended up not doing it until class time came. I think there is the kind of procrastination where someone is too busy doing other important things then they say they'll do it later but never really gets to it b/c they're still to busy doing whatever they are doing. And then there's the kind of procrastination where someone is just too lazy to even think about what they plan on doing and just don't do it until that someone runs out of time in the end to do it. I believe that there are good procrastinations, where someone has a decent excuse and there are bad procrastinations where someone is just lazy and prefer to do other, non-excusable things. To avoid/solve procrastination, I should just finish whatever I'm going to do, right when I get it in the first place.

That's it again for me! G-nites! ;]

BOB 2

Think of what are the cause(s) of procrastination and how they can affect you or someone you know. Are there different types of procrastination? What can you do to avoid/solve it?

This unit wasn't as long as I thought it would be. I did have some trouble doing the questions for homework but after that review day we had, I feel more confident. I'm not as worried about the test but you can never know enough I guess. I still need to understand how to find the derivative of a function and stuff like that. It's kind of fuzzy but I will get it before Friday! I decided to respond to Ashlynn's blog prompt for my BOB today =).

I think everyone procrastinates once in a while. I am definately one of those people who procrastinate a lot. I don't get home until very late most days because of extracurricular activities and when I get home, the only thing on my mind is sleep. Nothing else seems more important than much needed sleep! I end up leaving things for the last minute. What can I do to avoid it? I guess I should stay away from my room when I get home because it's the most distracting place for me. I should just do my homework before going into my room. Or do homework when I wake up (I wake up very early). I have about three hours to do what I have to do before school starts. Hopefully, I'll follow my own advice.

Everybody, good luck, study hard, and do well on your test on Friday!

BOB

Today's class went by pretty fast... All we did today was the derivative function pre- test and worked in groups, frantically trying to get all the answers finished. I believe that working with my group helped me a whole lot because they helped me see where I made my mistakes. Speaking of the pre-test, for me, it was overally straightforward... There were a few questions though in the quiz were I didn't quite understood what to do and what to answer. For instance, the last question of the pre-test. I totally guessed on that question... BUT... later in the day, Charlene and I went to Mr. K and asked for help on questions that we weren't too sure about. After we had asked for help, i finally understood the questions I was having trouble with!

My progress in this course is not of a slow or fast pace, but instead somewhere in between. I believe that my progress is beginning to increase in terms up how much i'm learning and understanding, each day I enter this class. Though, I think that my progress is beginning to decrease in terms of doing my exercise homework after it is assigned. I usually do my homework a day or even days after the day it is assigned. I should probably start doing my homework the day when it is assigned so that i won't forget the material we have learned during that day.

Blog Prompt:

A function can be defined symbolically, numerically and graphically. These representations are similar to each other b/c they all have the same meaning. Despite a function's different representaions, they still mean the same thing. People visually view those three representations as all being different, but in actual fact, they all mean and represent the same thing. Mr. K showed us a wooden block that very well structurizes how a funcion can be defined in 3 ways.

However, those three representations differ b/c of the way you see them through your eyes. For instance, you see a symbolic function as being an 'equation', a numerical function as being represented as a 'table of values' and a graphical function as being a 'graph'. These three representations differ in that manner. Another difference is how much easier it can be to use a certain represenation of a function, to solve a question, rather than using the others. For example, depending on the question, it may be easier to get the answer by doing a graphical representatin rather than doing a symbolic and numerical representation.

That's it for me...I should maybe go and do my 2nd BOB for the next test...yeah that's what i'll do... i've procrastinated long enough for this...laterz ;]

BOB

okay...derivatives...today's class of reviewing the techniques of finding the derivative really helped clear up some confusion I had. I had some trouble with the pre-test, I just didn't understand what to do or where to begin but my group cleared some of it up. I also found the long answer on the back of the pre-test difficult. I think I need more practise using the different techniques of finding the derivative because there are so many.

bob

wow this unit went by pretty fast lol. the thing that im having trouble with the most is going backwards from derivative graphs. its hard for me to go from the 2nd derivative and go to 1st derivative and then the parent function. another thing that confusses me is the roles that each of the derivatives play. like what the 1st and 2nd derivative determine. everything else is okay with me.

Blogon Blog #2

So in today's class we did our pre-test on the unit of derivatives. At first, I think I was just staring too hard at it, and completely blanked myself out. Then after some calm breathing to focus, it came to me. Studying for 4 hours yesterday paid off. In the end it was pretty simple! Although we still do not know what the answers on the back of the page are, our group got all the multiple choices on the front correct. I just hope we'll score with the long answer.

The only trouble I have is problems where it gives you a graph for no particular function, and then you're trying to find the derivative at for example lim h->0 f(3+h)-f(3) / h. I have no idea where to start with this type of question. If you do a direct substitution you get an undeterminite value. And if you find the value of f(3), then the problem is what's f(3+h) if you don't know what h is? If there was an equation where it tells you what f(x) is equal to then that I can do.

I'm in okay shape for the test on friday at this point. I can figure out what the graphs might possibly look like, from derivatives to its parent, and vice-versa, to also second-derivatives to its parent. And also expect a problem on the test that we hadn't really seen cause those sometimes come up (gut feeling). So do homework and be ready and good luck!

And to answer Ashylnn's blogging prompt. My motivation to procrastinate falls under everything that causes anyone to procrastinate. I know it's sad, but I would rather go cloud watching and do nothing anytime! (I hope that comment doesn't affect my marks =P). But things have to be done =].

scribe post

hey guys its charlene. i would of posted this earlier but then it got lost becuase i didn't save it lol. i cant put pictures of anything up because it won't let me. so please just bare with me..

alright so today we had our pre test on chapter 2 : derivative functions

1) if f(x) ln square root X, then the average rate of change of f on the intercal [3,7] is approxiamately:
..............a) 0.106 ........b) 0.189 ......c) 0.212...... d) 0.424 .....e) 0.847
- you graph the function on your calculator
- find the values for X=3 and X=7
-plot these values into the slope formula
-when you do this you get " 0.106 "


2) suppose the number of bacteria in a certain organism grows over time and the number, N(t), of bacteria (measured in thousands) at a time t is given by
N(t) = t(2+cost)^(4/3) + 3t
at approximately what time, t, in the first 10 days is the number of bacteria growing the fastest?
.............a) 5.0 .......b)5.6 .........c)5.12......... d) 5.18 .......e)5.24
-you can graph the function into you calculator
- you can use NDERIV to find the slopes at each value eg. 5.0 , 5.6
-the point with the largest slope is the answer, in this case it is 5.18


3) lim 2x^2+X-3
X->1 3x² -X-2
a) 2/3 b)3/2 c)1 d) 5.18 e)5.24
-factor out the function
lim (2X+3)(X-1)
X->1 (3X+2)(X-1)
-the (X-1) cancel out
-plug 1 into the remaining function and then you get an answer of 1


4) the graph of the second derivative of a function f is shown at right. which of the following is true?
I) the graph of f has an inflection point at X=-1
II) the f-graph is concave down on the interval (-1,3)
III) the graph of the derivative function f ' is increasing at X=1

a) 2/3 b)II only c) III only d) I and II only e) I, II, III


5) suppose a function f is defined so that it has derivatives:
f ' (x) = x²(x-1) and f ' (x) = x(3x - 2)
over what interval is the graph of f both increasing and conave up?
.................a) x less than o b) o<2/3.......c)2/3< x <1<>.......d) x>1......e) none of these


6) consider the following table of data for the function f
ill post the table later because it wouldn't let me sry

a) estimate f ' (5.2) as accurately as possible
you can use the slope formula and calculate the slope one unit before and after. then you find the average of those two slopes
.............(8.3-8.8) / (5.4 -5.2) = -2.5.......... (8.8-9.2) / (5.2-5.0) = -2
..................................................
.....................................................(2.5) + (-2) / 2
...........................................................=2.25

b) write the equation for the tangent line at x=5.2
- use the point slope formula
.............y-y1 = m (x-x1)
<sup>.............y-8.8 = -2.25 (x-5.2)
...................y = -2.25 (x-5.2) + 8.8

c) use your answer to part (b) to find approximately, f(5.26)
-just plug 5.26 into the formula</sup>
............y(5.26) -8.8 = 2.25 (5.26-5.2)
....................y(5.26) = -2.25(0.06) + 8.8
....................y(5.26) = 8.665

d) what is the sign of f '' (5.2)? explain your answer

the sign of f '' is NEGATIVE

-the slope of 5.0 to 5.2 is -2.0

-the slope of 5.2 to 5.4 is -2.5

-therefore the secant lines are decreasin

- therefore f ' is decreasing and f '' is less than zero on this this interval

THE NEXT SCRIBE IS JAN

Note

This is ashlynn again. This is just to remind you that my post showed up under Linger's post, instead of on top. I have no idea why! It did the same thing for my last post too ...

BOB

Ah.. procrastination. This is most definetly an area in which I have a huge problem.

The reason that I procrastinate is to avoid doing something I don't want to do; my philosophy is 'don't do today what can wait until tomorrow'. Unfortunately, when you have many commitments and you put off all of them, you will undoubtedly run out of time to accomplish everything. Of course, what would make the most sense is to sit down and finish something as soon as you get it. But I have a huge problem with sitting down and focusing. I can only finish something when I have JUST ENOUGH time left to finish it. If I start working on my math homework when I still have 5 hours of free time ahead, that homework will take 5 hours. Because I will stop after every question and get distracted by something. That something generally (on bad days) being THE DREADED INTERNET (dun dun DUN). However, on good days, I have (at least somewhat) valid reasons for putting something off. For example:

The reason I'm writing this BOB right now is
a) To avoid doing the supplementary problems
b) To avoid doing my physics homework

And the reason I haven't written the BOB earlier is
a) I typed up the agenda for tomorrow's meeting to avoid it
b) I reorganized all my files on Windows media for 2 hours to avoid it (yup.. this is where my 'bad' procrastinating took over)
c) I spent 20 mins writing down everything I have to do in my agenda to avoid doing all the things I was writing down.

Recently, I read this incredibly interesting article that describes a phenomenon called "Structured Procrastination" by John Perry. He describes my procrastination method to a T. I try to procrastinate in a productive way as often as possible, instead of just surfing the internet or going on msn. I think it is really quite unrealistic for me to try to stop procrastinating, so this is an honest way of improving myself and getting things done. If any of you think that making a schedule or locking yourself in an empty room after school with no way out is a bit too extreme, maybe this method can help you get things in a bit more control.

Here's the article: http://www.structuredprocrastination.com/
And thanks to the author, John Perry, for inspiring me to trick myself into working :)

And now, to start on that math homework...

BOB

Think of what are the cause(s) of procrastination and how they can affect you or someone you know. Are there different types of procrastination? What can you do to avoid/solve it?

Well this response is for ashlynn's post,=p. Hopefully i can respond to her blogging prompt and use it as my BOB. I think the causes of procrastination are :

1) Lazyness
2) Minds are on other things
3) Long day = fatigue
4) Lots of homework
5) Thinking you have a lot of time

i procrastinate too... for mostly everything, i am not sure why. if i could guess i would pick number 3 as my reason to procrastinate. I would rather stay awake when i get home and try to do my work at a turtles pace. My other option would be to take a nap then do my work, but thats just scary. It's scary because i might take a nap for 2+ hours and wake up at eight or something. Then i would have little time to do my HW. I think there might be different types of procrastination. There is procrastination avoidance, where you try to avoid doing something you really need to do. Then there is procrastination lazy, where you are just saying you will do something, but in reality too lazy.
So solutions to procrastination? Well, first you can get an agenda and plan your afternoon. Try to relax when you get home and eat. Mr.K said, "Set a time for yourself to do homework and do that everyday, to get your body used to doing homework." You can also try playing an instrument or playing your favorite sport. Your mind will get the stress off your head and you will get the blood flowing. Lastly, i think taking a hot shower can help, lol. The blood flows and loosens the clots in the brain, making you more relaxed.

SCRIBE POST DAY 29: REVIEW OF CHAPTER 2

Heey guys, it is Linger here once again at your service =) Like always, we had these questions on the board...

1. FIND THESE LIMITS:


Limits can be solved algebraically and technically (calculator). To solve limits algebraically, all you have to do is manipulate what you're given until you have an answer. *careful now... because numbers such as zero and two over zero are possible answers. When we get answers like zero over zero or infinity over infinity, we can use three different techniques to solve the limit...

• factor and reduce
• complex fractions
• radicals and rationalizing

When solving for limits technically, for example in d) we simply plug in the function on our Y= screen, graph it and look for the hole, or look at our table of values and determine where both sides of the function are approaching a certain value. In this case, the limit is 0. NOTE: TO TAKE AXES OFF, GO TO 2ND ZOOM, AND PICK AXES OFF. You should be able to see that there is a whole in the graph at zero, in your ZOOM 4 WINDOW.

2. GRAPH OF g:

Keep in mind that in order for the limit to exist, the right and left hand of the function have to be approaching the same place.

CONTINUITY Graphically, a function is continuous if you are able to draw the graph without lifting your pencil... this is ALWAYS true for all absolute value functions and many other functions. Formally, a function is continous on an interval [a,b] if for all x=a:

• I.....f(a) exists
• II ...lim...f(a) exists
• .....x->a
• III ..f(a) =.. lim....f(x)
  ..................x->a

We learned that the three types of discontinuity are removable, jump, and infinite. An example of an infinite discontinuity is the function of f(x) = 1 / (x-2 ). We also talked about the INTERMEDIATE VALUE THEOREM.

The EXTREME VALUE THEOREM also known as the EXISTENCE THEOREM :

In a closed interval, somewhere between a and b, an output bigger than all the others and an output smaller than all the others have to exist, in order for the function to be continuous.

3. A FUNCTION IS GIVEN BY;


* We didn't go over this question in depth, but on the right is what I got down. Basically, we factored the numerator and simplified. We used direct substitution and found that it is a removable discontinuity.

That brings us to the end of today's lesson. FOR HMWK; CHAPTER 2 SUPPLEMENTARY PROBLEMS ODD NUMBERS ONLY. We WILL be having our pre-test and test by the end of this week... STUDY HARD =D And Ashlynn, I CHOOSE YOU !!!!

Scribe Post Day 30

Today was basically a review of the Derivative Function.

The derivative function is the slope of the tangent lines of the parent function. When there is a zero, or root, of the derivative function, f'(x), we know that there will be a maximum or a minimum. This means that tangent lines are horizontal, with a slope of zero, on the parent function.

The blue graph is the derivative of the parent function.

F'(x) has two roots where x= -a, and x=a.

At x= -a, the graph of F '(x) is going from positive to negative, meaning that the parent function has a maximum at x= -a. The slopes of the tangent line on the parent function change from positive to negative after x = -a.

At x= a, F '(x) is going from negative to positive, meaning the the slopes of the tangent lines of the parent function at x = -a, are going from negative to positive. From that we know the parent function has a maximum at x = a.

___________________________________________________________________

The derivative function for this graph exists for everywhere on the graph of F(x) except at x = 0. At x = 0, there is a root but the derivative is undefined. This is because if you find the slope to the left and right of 0, you would end up with different slopes where one would be negative and the other is positive. At x = 0, there is a corner, making it impossible to find the derivative. It is not locally linear because no matter how much we zoom in on the graph there will always be a corner.

____________________________________________________________________

There are a number of ways to find the slope of a graph;

1) Use the slope program on your calculator.

2) Use your calculator to draw the tangent line at a point.

3) Use "nderive( " on your calculator.

Your calculator uses the difference quotient to find the slopes of the tangent lines. Your calculator also tries to use this at a point where the derivative should be undefined. If it gives a slope at that point then your calculator is lying.

4) You can use dy/dx, but before you use this you need the graph of the function on your calculator.

5) lim f(a+h) - f(a) / h

h->0

6) lim f(x+h) - F(x) / h You can use this to find the derivative function

h ->0

ex) f(x) = x ²

f(x+h) - F(x) / h = [ (x+h) ² - x² ] / h

=[ x² + 2xh +h² - x² ] / h

lim 2x +h

h->0

F '(x) = 2x

___________________________________________________________________

The blue graph, F(x), is the parent function.

The pink graph, F '(x), is the derivative function.

F '(x) is undefined at x = 0, it is negative to the left of 0. This means that the parent function has negative slopes for the tangent lines to the left of x = 0.

To the right of x = 0, F '(x) is positive. It must mean that the parent function has positive tangent lines to teh right of x=0

This is the second derivative, F "(x), of the derivative of the parent function, as seen above.

On F "(x), the graph is negative. This means that the parent function is concave down.

When F "(x) is positive, the parent function is concave up.

The point of inflection is at x = 0 or undefined on F "(x), because F '(x) is undefined at x = 0.

__________________________________________________________________

Continuity - a function is continuous if you can draw a graph without lifting your pencil.

A function is continuous if

I lim F(x) exists

x -> a

II F(a) exists

III lim F(x) = F(a)

Mr. K also said to look up the word stymieing .

stymieing - (1)a situation or problem presenting such difficulties as to

discourage or defeat any attempt to deal with or resolve it. (2) to hinder, block, or thwart.

Homework for tonight was to finish up the summary exercise. Tomorrow's scribe will be ... Charlene

Okay, I admit that I am not a fan of math, but this class truly helped me appreciate it more. I think it is because of how we are encouraged to work together, participate in class by asking questions, and to take part in the class blog. I feel more confident in this class than any other math class I've ever been in. Blogging has really helped me understand what we learned in class because it's like looking through someone else's eyes from class, and you see how you're similar and different.

Now answering the blogging prompt, a function can be described symbolically, numerically and graphically. Similarities are that they all are 3 ways or representing the same thing. Think of the block of wood Mr. K brings up all the time. The three ways are through a graph, an equation, and a table of values. The differences are; the graph gives you a visual representation of the function. You can see what is going on with the function. An equation is a symbolical representation of a function. What ever input you put in gives you an output. A table of values is the numerical representation. I can help you get an idea of how the graph will turn out, whether it is decreasing or increasing, or can be showing you a pattern.

One problem I have is actually taking the time to keep up with my homework. Procrastination is a BIG problem for me. I even stood BOB off billions of time... haha. I think it's because I suffer from EDD - EASILY DISTRACTED DISORDER! LOL You're all probably going to be upset with me for doing this but here it goes...

Mr. K said that he encouraged us to make our own BLOGGING PROMPT if we wish, so here's mine.

Blogging Prompt

Even the best and most gifted people can be procrastinators! You may even be reading this right now =P Even the incredibly talented Leonardo da Vinci was a procrastinator. It took him 20 years to finish painting the Mona Lisa. He also had some other unfinished projects. Although you can't blame the guy, he made so many contributions to so many different subjects like art and architecture, math, biology, physics, etc. It's so easy to be distracted with so many interests and jumping from idea to idea, project to project. This is probably how you feel about school or something else, and you're definitely not alone.

Think of what are the cause(s) of procrastination and how they can affect you or someone you know. Are there different types of procrastination? What can you do to avoid/solve it?

Well that's all, good luck with Project Emancipation Procrastination ^-^ Feel free to add comments or anything you want to the prompt if it helps to explain it better or something.

Scribe Post: Continuity

We had a substitute and we were assigned 2.8: Continuity in the textbook. We had to read and do all the odd numbers.

I'm pretty confused about Continuity among other things so all I can suggest is to make sense of the textbook. If I understand it then I'll blog about what I know.

We should go over this tomorrow (Monday) so that we understand it before our upcoming test.

Don't forget your bobs!

Yay. This is the last part in the derivatives unit. Well, that's what the textbook tells me. The next scribe will be Linger. I think she's the only one left for cycle 2.

Scribe Post: Day 27, Derivative Function

Hey, hey, hey! Class started off with.....A QUIZ!!! WHOO HOO! Here's the questions and solutions:

1. Suppose f(x) = √x.

a) Calculate the average rate of change between x = 9 and x = 9.1.

f(9.1) - f(9) / 9.1 - 9.0 = average rate of change
√9.1 - √9 / 0.1 = 0.1662 (Store the answer from your calc in A)

b) Calculate the average rate of change between x = 8.9 and x = 9.0.

f(9) - f(8.9) / 9 - 8.9 = average rate of change
√9 - √8.9 / 0.1 = 0.1671 (Store the answer from your calc in B)

c) Use your answers above to approximate the instantaneous rate of change at x = 9.0.

Take the answers from questions A and B:
(A+B) / 2 = 0.16666

NOTE: You must have your answers for A) and B) to four decimal places, you don't start seeing a change between the two answers until four decimal places. Marks normally aren't given out if your answers aren't to four decimal places. When calculating the answer to C) in your calculator, you must have brackets around A + B or the calculator will calculate B / 2 then + A into the solution.


2. A ball is dropped from a height of 400 ft and falls towards the earth in a straight line. In t seconds the ball drops a distance of d = 16t² feet.

a) How many seconds after release does the ball hit the ground?

The ball is dropped from a height of 400 ft, therefore the ball must travel 400 ft before it hits the ground:

400 = 16t²
400 / 16 = t²
t = 5

b) What is the average velocity of the ball during the time it is falling?

0 - 400 / 5 - 0 = -80 ft/sec

The reason why we take 400 and subtract it from 0 and not the other way around is because the ball is falling down not falling up, so the velocity is increasing in a negative manner.

c) Estimate the instantaneous velocity of the ball when it hits the ground.

160 ft/sec

There are many methods of finding this answer. You can graph it and keep zooming into that point and use the "SLOPE" program, you can estimate by using the symetric difference quotient and others. Unfortunately my mind is mashed right now and I can't recall any other from the top of my head. =S


3. An object travels in such a way that its position at various points in time is given in the table:

a) Find the average velocity of the object between t = 1.3 and t = 1.9.

5.3 - 5.1 / 1.9 - 1.3 =
0.2 / 0.6 = 1/3

b) Estimate the instantaneous velocity at t = 1.9. Show work.

5.3 - 5.6 / 1.9 - 1.8 =
-0.3 / 0.1 = -3


While Mr. K was giving us the solutions to this quiz he talked about the exam for the course. Here's a rough outline of the exam:

• consists of two sections, multiple choice and long answer.
• each section has a non-calculator part and a calculator part.
• multiple choice has 28 questions non-calculator (50 min) and 17 calculator questions (50 min).
• long answer has 3 calculator questions (50 min) and 3 questions non-calculator (50 min).
• the questions and sections come in the order I just explained.
• the exam itself is a MARATHON 4 HOURS from 8 a.m. to 12 p.m.

Now onto the topic we talked about in class....or at least what I managed to write down of it. =S "Limits"

We started with a function f(x) = 2x - 1: (Note: graph may be up tomorrow, it's too late for me honestly =S)

We were given lim x->2 f(x) which is, lim x->2 2x - 1. If you were to try and find x = 2 the answer would come up to be 3. But the lim x->2 means you have a value very close to 2 your input and a value very close to 3 your output, but it never actually gets to the point (2,3). I'm not entirely sure if what I just explained made any sense I made a lot of point form notes and I'm trying to work from it I apologize now if any of my information is incorrect or has no value to it.

We were then given (x² - 1) / (x - 1) and we graphed it on our calculators. When the graph was shown in a "Zoom Standard" window it looked like just an ordinary straight line. But when you look at it in a "Zoom Decimal" window, you see that the line in fact has a HOLE in it! The reason for this is because (x² - 1) factors and reduces. The hole in the graph is at x = 1 because there is no value for that. When you look at the table there seems to be a limit at x = 1. The y values get closer and closer to 2 but never really gets there. Therefore x can never equal 1 cause of the limit.

lim x->1 (x²- 1) / (x - 1) = (x - 1)(x + 1) / (x - 1) = (x + 1)

This is were my notes get a little confusing =S. Mr. K started talking about dividing by 0 and why it's not allowed. When you multiple 2 by 3 you get 6 and when you divide 6 by 3 you get 2, so when you multiple 2 by 0 you get 0 and when you divide 0 by 0 you get 2? So basically 0/0 can give you any number? We call this "indeterminate". If you divide 5 by 1 you get 5, if you divide 5 by 0.1 you get 50, if you divide 5 by 0.01 you get 500 and so on. As your denominator gets smaller and your numerator stays the same the value it generates increases until ∞, which isn't a number therefore you can't divide by 0.

This is were my notes seem to be back on track =S. We graphed abs(x) / x and when you go "Trace" x=0 you see that y has no value. Looking on the table there's two limits, these are called the right hand limit and the left hand limit. The right hand limit is at 1 and the left hand limit is at -1. Numerically it's written like this:

lim x->0+ abs(x) / x lim x->0- abs(x) / x

If you were to have lim x->0 abs(x) / x, this would work if on the graph the above limits met at the same place, however they don't so the value does not exist.

Homework for tonight, or should I say earlier was I believe EXERCISE 2.7 on The Limit of a Function. I think it was just odd number questions only. There was also suppose to be a link or something of some sort to this derivative quiz on this website but last time I checked it hadn't been up yet.

Once again I apologize if any of this seems jibberish to you, please feel welcome to comment and tell me every I missed for this class (which is probably a lot) and the next scribe will be Lindsay. It was an easy choice, she told me to pick her and me being the nice guy I am had to agree =D