Scribe Post: Day 64

Hi ya'll! This is Jann and I'll be your scribe for today. XD

Today, we continued our discussion on Antiderivatives. Here we go...

Last Friday, we started our discussion and we came up with the first antiderivative rule.
An antiderivative is basically just DIFFERENTIATING the function backwards. The rule for finding the FIRST derivative from the PARENT function has been reversed.

For example: Find the antiderivative of f'(x) = x.

Applying the rules for the antiderivative, we came up with the answer. If your wondering where the "C" came from, good for you! C is an unknown constant in the parent function. We don't have any evidences that a constant exists within the parent function.

Note: Remember that the derivative of any constant is 0.

What if...

For this example, we were given a point in the graph, (1,2). Using this information, we can identify the unknown constant, "C", by substituting the values into the parent function.
Then, we discussed the other antiderivative rules.Note: Remember that the derivative of cosX is -sinX. Therefore the "antiderivative" of "sinX" is the negative of "cosX".

Note: "Anything you can do, you can Undo..." (Mr.K) Every single derivative rule we have learned has an equivalent antiderivative rule!

How do we know if the antiderivative is correct? We can DIFFERENTIATE the antiderivative we came up with and see if the answer is the given question. It is the same as checking a set of factors by distributing the factors and see if the answer is the same as the given function.

For example:

f(x) = 2x^2 - 4x
= 2x(x-2)

Note: "Any high school level functions CAN be differentiated or antidifferentiated..." (Mr.K)

Then, we reviewed the "Definite Integral".
If you're wondering why antiderivatives have a stretched "s" like a definite integral, congratulations! The format of the antiderivative itself is formally known as an "Indefinite Integral". It's the opposite of a definite integral because it has NO limits/intervals (the number at the 2 ends of the "s").

Here are some examples we discussed:

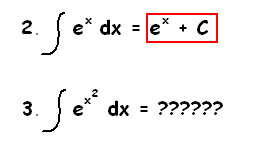
If your wondering why number 3 has no answer, THERE IS NO ANSWER TO IT for now. No one knows the antiderivative for this function. If anyone answers this, that lucky person will be famous and his/her name will be written in the history books! Cool eh? XD

Lastly, we discussed this concept:
Correction: "no inflection points [f"(x) not equal 0]"

We explored the concept of this function. We found the first and second derivatives. Then, we used the first derivative to find the critical number, -b/2. Using this critical number, we can explore the graph of the function. Using three values, b>0, b=0, b<0,>

Whew... that took a while... XD. Anyways, I hope I covered all the things we did today.

Reminders: Pre-test tomorrow! Put up your BOB's before Wednesday.

Oh and BTW, Manny is scribe for tomorrow. XD